Business Management Courses
|
|||
|
|
|||
|
|
Business Management CoursesBusiness Management Training Courses Online
International Institute of Management Training Courses in Las Vegas USA Accelerated
Management Workshops in Las Vegas, USA
Online Distance Learning (ODL) Courses (Worldwide Access)
Directory of Management Training Courses
Management Consulting Courses Management Training Courses Online Business Schools Free Open Courses Business Schools Online Business Management Courses CEO Magazine CEO Library Executive Networking CEO Books Review of MBA Programs Ranking of World's Best MBA Programs Online MBA Ranking of Top MBA Programs Online Executive MBA Programs Accelerated MBA Programs Management Science : Operations Research and Management Decision
Book Start
Management Course Introduction to Operational Research & Management Decision Course
Management Course 1: Introduction to Operational Research Introduction to Operational Research This teaching module is designed to be an entertaining and representative introduction to the subject of Operational Research. It is divided into a number of sections each covering different aspects of OR. What is Operational Research? This looks at the characteristics of Operational Research, how you define what OR is and why organizations might use it. It considers the scientific nature of OR and how it helps in dealing with problems involving uncertainty, complexity and conflict. OR is the representation of real-world systems by mathematical models together with the use of quantitative methods (algorithms) for solving such models, with a view to optimising. Battle of the Atlantic Considers the origins of OR in the British military and looks at how OR helped to ensure the safety of merchant ships during the "Battle of the Atlantic" in World War II. Introduction to ORTerminologyThe British/Europeans refer to "operational research", the Americans to "operations research" - but both are often shortened to just "OR" (which is the term we will use). Another term which is used for this field is "management science" ("MS"). The Americans sometimes combine the terms OR and MS together and say "OR/MS" or "ORMS". Yet other terms sometimes used are "industrial engineering" ("IE") and "decision science" ("DS"). In recent years there has been a move towards a standardisation upon a single term for the field, namely the term "OR". BooksThere are many books on OR available in the college library and you should not need to buy any books. If you do find you need a book then I recommend: J.K.Sharma: Operations Research (Theory and Application) N.D.Vohra: Quantitative Techniques in Management
JournalsOR is a new field which started in the late 1930's and has grown and expanded tremendously in the last 30 years (and is still expanding). As such the academic journals contain many useful articles that reflect state of the art applications of OR. We give below a selection of the major OR journals.
The first seven of the above are mainly theoretical whilst the eighth (Interfaces) concentrates upon case studies. All of these journals are available in the college library so have a browse through them to see what is happening in state of the art OR. Note here that my personal view is that in OR, as in many fields, the USA is the country that leads the world both in the practical application of OR and in advancing the theory (for example, the American OR conferences have approximately 2500 participants, the UK OR conference has 300). One thing I would like to emphasise in relation to OR is that it is (in my view) a subject/discipline that has much to offer in making a real difference in the real world. OR can help you to make better decisions and it is clear that there are many, many people and companies out there in the real world that need to make better decisions. I have tried to include throughout OR-Notes discussion of some of the real-world problems that I have personally been involved with.
History of OROR is a relatively new discipline. Whereas 70 years ago it would have been possible to study mathematics, physics or engineering (for example) at university it would not have been possible to study OR, indeed the term OR did not exist then. It was really only in the late 1930's that operational research began in a systematic fashion, and it started in the UK. As such I thought it would be interesting to give a short history of OR and to consider some of the problems faced (and overcome) by early OR workers. Whilst researching for this short history I discovered that history is not clear cut, different people have different views of the same event. In addition many of the participants in the events described below are now elderly/dead. As such what is given below is only my understanding of what actually happened. Note: some of you may have moral qualms about discussing what are, at root, more effective ways to kill people. However I cannot change history and what is presented below is essentially what happened, whether one likes it or not. 1936Early in 1936 the British Air Ministry established Bawdsey Research Station, on the east coast, near Felixstowe, Suffolk, as the centre where all pre-war radar experiments for both the Air Force and the Army would be carried out. Experimental radar equipment was brought up to a high state of reliability and ranges of over 100 miles on aircraft were obtained. It was also in 1936 that Royal Air Force (RAF) Fighter Command, charged specifically with the air defense of Britain, was first created. It lacked however any effective fighter aircraft - no Hurricanes or Spitfires had come into service - and no radar data was yet fed into its very elementary warning and control system. It had become clear that radar would create a whole new series of problems in fighter direction and control so in late 1936 some experiments started at Biggin Hill in Kent into the effective use of such data. This early work, attempting to integrate radar data with ground based observer data for fighter interception, was the start of OR. 1937The first of three major pre-war air-defence exercises was carried out in the summer of 1937. The experimental radar station at Bawdsey Research Station was brought into operation and the information derived from it was fed into the general air-defense warning and control system. From the early warning point of view this exercise was encouraging, but the tracking information obtained from radar, after filtering and transmission through the control and display network, was not very satisfactory. 1938In July 1938 a second major air-defense exercise was carried out. Four additional radar stations had been installed along the coast and it was hoped that Britain now had an aircraft location and control system greatly improved both in coverage and effectiveness. Not so! The exercise revealed, rather, that a new and serious problem had arisen. This was the need to coordinate and correlate the additional, and often conflicting, information received from the additional radar stations. With the out-break of war apparently imminent, it was obvious that something new - drastic if necessary - had to be attempted. Some new approach was needed. Accordingly, on the termination of the exercise, the Superintendent of Bawdsey Research Station, A.P. Rowe, announced that although the exercise had again demonstrated the technical feasibility of the radar system for detecting aircraft, its operational achievements still fell far short of requirements. He therefore proposed that a crash program of research into the operational - as opposed to the technical - aspects of the system should begin immediately. The term "operational research" [RESEARCH into (military) OPERATIONS] was coined as a suitable description of this new branch of applied science. The first team was selected from amongst the scientists of the radar research group the same day. 1939In the summer of 1939 Britain held what was to be its last pre-war air defence exercise. It involved some 33,000 men, 1,300 aircraft, 110 antiaircraft guns, 700 searchlights, and 100 barrage balloons. This exercise showed a great improvement in the operation of the air defence warning and control system. The contribution made by the OR teams was so apparent that the Air Officer Commander-in-Chief RAF Fighter Command (Air Chief Marshal Sir Hugh Dowding) requested that, on the outbreak of war, they should be attached to his headquarters at Stanmore in north London. Initially, they were designated the "Stanmore Research Section". In 1941 they were redesignated the "Operational Research Section" when the term was formalised and officially accepted, and similar sections set up at other RAF commands. 1940On May 15th 1940, with German forces advancing rapidly in France, Stanmore Research Section was asked to analyse a French request for ten additional fighter squadrons (12 aircraft a squadron - so 120 aircraft in all) when losses were running at some three squadrons every two days (i.e. 36 aircraft every 2 days). They prepared graphs for Winston Churchill (the British Prime Minister of the time), based upon a study of current daily losses and replacement rates, indicating how rapidly such a move would deplete fighter strength. No aircraft were sent and most of those currently in France were recalled.
1941 onwardIn 1941 an Operational Research Section (ORS) was established in Coastal Command which was to carry out some of the most well-known OR work in World War II. The responsibility of Coastal Command was, to a large extent, the flying of long-range sorties by single aircraft with the object of sighting and attacking surfaced U-boats (German submarines). Amongst the problems that ORS considered were:
Here the problem was that in a squadron each aircraft, in a cycle of approximately 350 flying hours, required in terms of routine maintenance 7 minor inspections (lasting 2 to 5 days each) and a major inspection (lasting 14 days). How then was flying and maintenance to be organised to make best use of squadron resources? ORS decided that the current procedure, whereby an aircrew had their own aircraft, and that aircraft was serviced by a devoted ground crew, was inefficient (as it meant that when the aircraft was out of action the aircrew were also inactive). They proposed a central garage system whereby aircraft were sent for maintenance when required and each aircrew drew a (different) aircraft when required. The advantage of this system is plainly that flying hours should be increased. The disadvantage of this system is that there is a loss in morale as the ties between the aircrew and "their" plane/ground crew and the ground crew and "their" aircrew/plane are broken.
This is held by some to be the most strategic contribution to the Management Course of the war made by OR (as the aircraft and pilots saved were consequently available for the successful air defense of Britain, the Battle of Britain). The first use of OR techniques in India, was in the year] 1949 at Hyderabad, where at the Regional Research Institute, an independent operations research unit was set-up. To identify evaluate and solve the problems related to planning, purchases and proper maintenance of stores, an operations research unit was also setup at the Defence Science Laboratory use of OR tools and techniques was done during India's second five year Plan in demand forecasting and suggesting the most suitable scheme which would lead to the overall growth and the development of the economy. Even today, Planning Commission utilises some. of these techniques in framing policies and sector-wise performance evaluation. In 1953, at the Indian Statistical Institute (Calcutta). A self-sufficient operations research unit was established for the purpose of national planning & survey. OR Society of India was folined in 1957 which publishes journal titled "Of search". Many big and prominent business & industrial houses are using extensively the tools of OR for the optimum utilisation of precious and scarce resources available to them. This phenomenon is not limited to the private sector only. Even good companies in the public sector ("Nav Ratnas") are reaping the benefits of fully functional sound OR units. Example of such corporate, both private & public are: SAIL, BHEL, NTPC, Indian Railways, Indian Airlines, Air-India, Hindustan Lever, TELCO & TISCO etc. Textile companies engaged in the process of manufacture of various types of fabrics use some of the tools of OR like lenear programming & PERT in their blending, dyeing and other manufacturing operations. Various other Indian companies are .employing OR techniques for solving problems pertaining to various spheres of activities, as diverse as advertising, sales promotion, inspection, quality control, staffing, personnel, investment & production planning, etc. These organisations are not only employing the operations research techniques and analysis on a short-term trouble-shooting basis but also for ong-range strategic planning.
Basic OR conceptsDefinitionSo far we have avoided the problem of defining exactly what OR is. In order to get a clearer idea of what OR is we shall actually do some by considering the specific problem below and then highlight some general Management Courses and concepts from this specific example. Two Mines CompanyThe Two Mines Company own two different mines that produce an ore which, after being crushed, is graded into three classes: high, medium and low-grade. The company has contracted to provide a smelting plant with 12 tons of high-grade, 8 tons of medium-grade and 24 tons of low-grade ore per week. The two mines have different operating characteristics as detailed below. Mine Cost per day (£'000) Production (tons/day) High Medium Low X 180 6 3 4 Y 160 1 1 6 How many days per week should each mine be operated to fulfil the smelting plant contract? Note: this is clearly a very simple (even simplistic) example but, as with many things, we have to start at a simple level in order to progress to a more complicated level. GuessingTo explore the Two Mines problem further we might simply guess (i.e. use our judgement) how many days per week to work and see how they turn out.
This does not seem like a good guess as it results in only 7 tonnes a day of high-grade, insufficient to meet the contract requirement for 12 tonnes of high-grade a day. We say that such a solution is infeasible.
This seems like a better guess as it results in sufficient ore to meet the contract. We say that such a solution is feasible. However it is quite expensive (costly). Rather than continue guessing we can approach the problem in a structured logical fashion as below. Reflect for a moment though that really we would like a solution which supplies what is necessary under the contract at minimum cost. Logically such a minimum cost solution to this decision problem must exist. However even if we keep guessing we can never be sure whether we have found this minimum cost solution or not. Fortunately our structured approach will enable us to find the minimum cost solution.
Two Mines solutionWhat we have is a verbal description of the Two Mines problem. What we need to do is to translate that verbal description into an equivalent mathematical description. In dealing with problems of this kind we often do best to consider them in the order:
We do this below and note here that this process is often called formulating the problem (or more strictly formulating a mathematical representation of the problem). (1) Variables These represent the "decisions that have to be made" or the "unknowns". Let x = number of days per week mine X is operated y = number of days per week mine Y is operated Note here that x >= 0 and y >= 0. (2) Constraints It is best to first put each constraint into words and then express it in a mathematical form.
Ore High 6x + 1y >= 12 Medium 3x + 1y >= 8 Low 4x + 6y >= 24 Note we have an inequality here rather than an equality. This implies that we may produce more of some grade of ore than we need. In fact we have the general rule: given a choice between an equality and an inequality choose the inequality. For example - if we choose an equality for the ore production constraints we have the three equations 6x+y=12, 3x+y=8 and 4x+6y=24 and there are no values of x and y which satisfy all three equations (the problem is therefore said to be "over-constrained"). For example the values of x and y which satisfy 6x+y=12 and 3x+y=8 are x=4/3 and y=4, but these values do not satisfy 4x+6y=24. The reason for this general rule is that choosing an inequality rather than an equality gives us more flexibility in optimising (maximising or minimising) the objective (deciding values for the decision variables that optimise the objective).
x <= 5 y <= 5 Constraints of this type are often called implicit constraints because they are implicit in the definition of the variables. (3) Objective Again in words our objective is (presumably) to minimise cost which is given by 180x + 160y Hence we have the complete mathematical representation of the problem as: minimise 180x + 160y subject to 6x + y >= 12 3x + y >= 8 4x + 6y >= 24 x <= 5 y <= 5 x,y >= 0
There are a number of points to note here:
DiscussionConsidering the Two Mines example given above:
Essentially an algorithm (for a particular model) is a set of instructions which, when followed in a step-by-step fashion, will produce a numerical solution to that model. You will see some examples of algorithms later in this Management Course..
Hence we have a definition of OR as: OR is the representation of real-world systems by mathematical models together with the use of quantitative methods (algorithms) for solving such models, with a view to optimising. One thing I wish to emphasise about OR is that it typically deals with decision problems. You will see examples of the many different types of decision problem that can be tackled using OR. We can also define a mathematical model as consisting of:
PhilosophyIn general terms we can regard OR as being the application of scientific methods/thinking to decision making. Underlying OR is the philosophy that:
Indeed it can be argued that although OR is imperfect it offers the best available approach to making a particular decision in many instances (which is not to say that using OR will produce the right decision). Often the human approach to decision making can be characterised (conceptually) as the "ask Fred" approach, simply give Fred ('the expert') the problem and relevant data, shut him in a room for a while and wait for an answer to appear. The difficulties with this approach are:
You can form your own judgement as to whether OR is better than this approach or not.
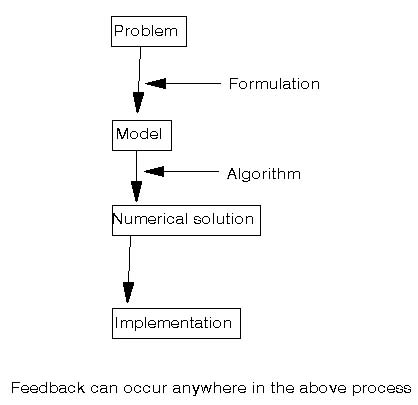
Phases of an OR projectDrawing on our experience with the Two Mines problem we can identify the phases that a (real-world) OR project might go through. 1. Problem identification
2. Formulation as a mathematical model It may be that a problem can be modelled in differing ways, and the choice of the appropriate model may be crucial to the success of the OR project. In addition to algorithmic considerations for solving the model (i.e. can we solve our model numerically?) we must also consider the availability and accuracy of the real-world data that is required as input to the model. Note that the "data barrier" ("we don't have the data!!!") can appear here, particularly if people are trying to block the project. Often data can be collected/estimated, particularly if the potential benefits from the project are large enough. You will also find, if you do much OR in the real-world, that some environments are naturally data-poor, that is the data is of poor quality or nonexistent and some environments are naturally data-rich. As examples of this church location study (a data-poor environment) and an airport terminal check- in desk allocation study (a data-rich environment). This issue of the data environment can affect the model that you build. If you believe that certain data can never (realistically) be obtained there is perhaps little point in building a model that uses such data. 3. Model validation (or algorithm validation) Model validation involves running the algorithm for the model on the computer in order to ensure:
4. Solution of the model Standard computer packages, or specially developed algorithms, can be used to solve the model (as mentioned above). In practice, a "solution" often involves very many solutions under varying assumptions to establish sensitivity. For example, what if we vary the input data (which will be inaccurate anyway), then how will this effect the values of the decision variables? Questions of this type are commonly known as "what if" questions nowadays. Note here that the factors which allow such questions to be asked and answered are:
5. Implementation This phase may involve the implementation of the results of the study or the implementation of the algorithm for solving the model as an operational tool (usually in a computer package). In the first instance detailed instructions on what has to be done (including time schedules) to implement the results must be issued. In the second instance operating manuals and training schemes will have to be produced for the effective use of the algorithm as an operational tool. It is believed that many of the OR projects which successfully pass through the first four phases given above fail at the implementation stage (i.e. the work that has been done does not have a lasting effect). As a result one topic that has received attention in terms of bringing an OR project to a successful conclusion (in terms of implementation) is the issue of client involvement. By this is meant keeping the client (the sponsor/originator of the project) informed and consulted during the Management Course of the project so that they come to identify with the project and want it to succeed. Achieving this is really a matter of experience. A graphical description of this process is given below.
The phases that a typical OR project might go through are:
We would be looking for a discussion of these points with reference to one particular problem. Example OR projectsNot all OR projects get reported in the literature (especially OR projects which fail). However to give you an idea of the areas in which OR can be applied we give below some abstracts from papers on OR projects that have been reported in the literature (all projects drawn from the journal Interfaces). Note here that, at this stage of the Management Course, you will probably not understand every aspect of these abstracts but you should have a better understanding of them by the end of the Management Course.
Critical to an airline's operation is the effective use of its reservations inventory. American Airlines began research in the early 1960's in managing revenue from this inventory. Because of the problem's size and difficulty, American Airlines Decision Technologies has developed a series of OR models that effectively reduce the large problem to three much smaller and far more manageable subproblems: overbooking, discount allocation and traffic management. The results of the subproblem solutions are combined to determine the final inventory levels. American Airlines estimates the quantifiable benefit at $1.4 billion over the last three years and expects an annual revenue contribution of over $500 million to continue into the future. Yield management is also sometimes referred to as capacity management. It applies in systems where the cost of operating is essentially fixed and the focus is primarily, though not exclusively, on revenue maximisation. For example all transport systems (air, land, sea) operating to a fixed timetable (schedule) could potentially benefit from yield management. Hotels would be another example of a system where the focus should primarily be on revenue maximisation. To give you an illustration of the kind of problems involved in yield management suppose that we consider a specific flight, say the 4pm on a Thursday from Chicago O'Hare to New York JFK. Further suppose that there are exactly 100 passenger seats on the plane subdivided into 70 economy seats and 30 business class seats (and that this subdivision cannot be changed). An economy fare is $200 and a business class fare is $1000. Then a fundamental question (a decision problem) is : How many tickets can we sell ? One key point to note about this decision problem is that it is a routine one, airlines need to make similar decisions day after day about many flights. Suppose now that at 7am on the day of the flight the situation is that we have sold 10 business class tickets and 69 economy tickets. A potential passenger phones up requesting an economy ticket. Then a fundamental question (a decision problem) is : Would you sell it to them ? Reflect - do the figures given for fares $200 economy, $1000 business affect the answer to this question or not ? Again this decision problem is a routine one, airlines need to make similar decisions day after day, minute after minute, about many flights. Also note that in this decision problem an answer must be reached quickly. The potential passenger on the phone expects an immediate answer. One factor that may influence your thinking here is consider certain money (money we are sure to get) and uncertain money (money we may, or may not, get). Suppose now that at 1pm on the day of the flight the situation is that we have sold 30 business class tickets and 69 economy tickets. A potential passenger phones up requesting an economy ticket. Then a fundamental question (a decision problem) is : Would you sell it to them ?
With operations extending from the east coast to Hawaii, GTE is the largest local telephone company in the United States. Even before its 1991 merger with Contel, GTE maintained more than 2,600 central offices serving over 15.7 million customer lines. It does extensive planning to ensure that its $300 million annual investment in customer access facilities is well spent. To help GTE Corporation in a very complex task of planning the customer access network, GTE Laboratories developed a decision support tool called NETCAP that is used by nearly 200 GTE network planners, improving productivity by more than 500% and saving an estimated $30 million per year in network construction costs.
GE Capital provides credit card services for a consumer credit business exceeding $12 billion in total outstanding dollars. Its objective is to optimally manage delinquency by improving the allocation of limited collection resources to maximize net collections over multiple billing periods. We developed a probabilistic account flow model and statistically designed programs to provide accurate data on collection resource performance. A linear programming formulation produces optimal resource allocations that have been implemented across the business. The PAYMENT system has permanently changed the way GE Capital manages delinquent consumer credit, reduced annual losses by approximately $37 million, and improved customer goodwill. Note here that GE Capital also operates in the UK. Operational research example 1987 UG examManaging director of the company started as a tea-boy 40 years ago and rose through the ranks of the company (without any formal education) to his present position. He believes that all a person needs to succeed in business are (innate) ability and experience. What arguments would you use to convince him that the decision-making techniques dealt with in this Management Course are of value? SolutionThe points that we would expect to see in an answer include:
|
Business Management Websites
|
|